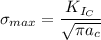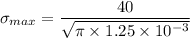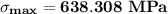Answer:
Step-by-step explanation:
For any large steel plate with an infinite length and width and a center through the crack under tension, the stress intensity factor can be expressed as:

where;
a = 2.5 mm/2
a = 1.25 mm
a = 1.25 × 10⁻³ m
Therefore; the maximum stress in tension capacity can be computed as;