Question

Solve the proportion below. 7/x = 19/144

Answer 1

The option B) 42 is the correct answer.

Step-by-step explanation:

Concept :

Here, we will use the below following steps to find a solution using the transposition method:

• Step 1 :- we will Identify the variables and constants in the given simple equation.
• Step 2 :- then we Simplify the equation in LHS and RHS.
• Step 3 :- Transpose or shift the term on the other side to solve the equation further simplest.
• Step 4 :- Simplify the equation using arithmetic operation as required that is mentioned in rule 1 or rule 2 of linear equations.
• Step 5 :- Then the result will be the solution for the given linear equation.

`\begin{gathered}\end{gathered}`

Question :

Solve the proportion below.

`{\implies{\sf{(7)/(x) = (19)/(114)}}}`

`\begin{gathered}\end{gathered}`

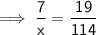
Solution :

`{\implies{\sf{(7)/(x) = (19)/(114)}}}`

`{\implies{\sf{19 * x = 114 * 7}}}`

`{\implies{\sf{19x = 798}}}`

`{\implies{\sf{x = (798)/(19)}}}`

`{\implies{\sf{x = \cancel{(798)/(19)}}}}`

`{\implies{\sf{x = 42}}}`

`\star{\underline{\boxed{\sf{\purple{x = 42}}}}}`

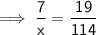
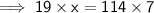
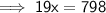
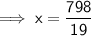
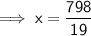
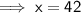
Hence, the value of x is 42.

`\rule{300}{2.5}`

Answer 2

`\boxed{\boxed{\tt B)\: 42}}`

Explanation:

`\tt \cfrac{7}{x}=\cfrac{19}{114}`

Cross multiply:

(Multiply the numerator in the first fraction times the denominator in the second fraction, then multiply the numerator of the second fraction times the number in the denominator of your first fraction.)

`\longmapsto\tt 798=x* \:19`

Switch sides:

`\longmapsto\tt x* \:19=798`

Divide both sides by 19:

`\longmapsto\tt \cfrac{x* \:19}{19}=\cfrac{798}{19}`

Divide 798 by 19 = 42

`\longmapsto\tt x=\cfrac{798}{19}`

`\hookrightarrow \tt x=42`

______________________________