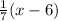Answer:

Explanation:
Two points (1, -1) and (0, 6) are lying on the given line.
Rule to find the coordinates of a point by the rotation of 90° clockwise is,
(x, y) → (y, -x)
Therefore, image points of (1, -1) and (0, 6) will be,
(1, -1) → (-1, -1)
(0, 6) → (6, 0)
Slope of the line passing through two points
 and
and
 is given by,
is given by,
m =
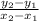
Therefore, slope of the line passing through (-1, -1) and (6, 0) will be,
m =
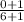 =
=

Equation of the line passing through (6, 0) and slope =

y - y' = m(x - x')
y - 0 =