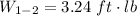Question

(based on 15-103 in the text) At an initial instant, a 4-lb ball B is traveling around in a circle of radius r1 = 3 ft with a speed (vB)1 = 4.8 ft/s. The attached cord is then pulled down through the hole with a constant speed vr = 2.2 ft/s. a. Determine the ball's speed at the instant r2 = 2 ft. Neglect friction and the size of the ball. Note that particle path is no longer of constant radius, and the particle has velocity components in both tangential and radial directions. b. How much work is done to pull down the cord from the initial instant to the instant when r2 = 2 ft? Neglect friction and the size o

Answer 1

a

`v_r =8.65 \ ft/s`

b

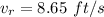
`W_(1-2) =  3.24 \  ft \cdot lb`

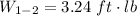
Step-by-step explanation:

From the question we are told that

The mass of the ball is
`m  =  4 \  lb`

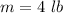
The radius is
`r= 3 \  ft`

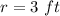
The speed is
`v_B_1  =  4.8 \ ft /s`

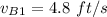
The speed of the attached cord is
`v_c =2.2 \  ft`

The position that is been considered is
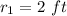`r_1 =  2 \  ft`h

Generally according to the law of angular momentum conservation

`L_a =  L_b`

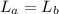
Here
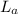`L_a` is the initial momentum of the ball which is mathematically represented as

`L_a  =  m*  v_B_1 *  r`

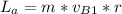
while

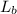`L_b` is the momentum of the ball at r = 2 ft which is mathematically represented as

`L_a  =  m*  v_B_2 *  r_1`

So

`m*  v_B_1 *  r = m*  v_B_2 *  r_1`

=>
`4.8 *  3 =  v_B_2 *  2`

=>
`v_B_2 =  7.2 \  ft/s`

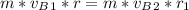
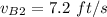
Generally the resultant velocity of the ball is

`v_r = √(v_B_2^2 + v_B_1^2   )`

=>
`v_r = √(7.2^2 + 4.8^2   )`

=>
`v_r =8.65 \ ft/s`

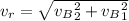
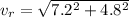
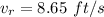
Generally according to equation for principle of work and energy we have that

`K_1 + \sum W_(1-2) = K_2`

Here
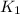`K_1` is the initial kinetic energy of the ball which is mathematically represented as

`K_1  =  (1)/(2)  *  m* v_B_1^2`

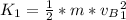
While
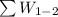`\sum W_(1-2)` is the sum of the total workdone by the ball

and
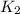`K_2` is the final kinetic energy of the ball which is mathematically represented as
`K_2  =  (1)/(2)  *  m* v_r^2`

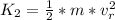
So

`\sum W_(1-2) =  (1)/(2)  *  m  (v_r^2  -  v_B_1^2)`

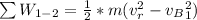
Here m is the mass which is mathematically represented as

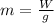`m = (W)/(g)` here W is the weight in lb and g is the acceleration due to gravity which is
`g =  32 \ ft/s^2`

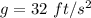
So

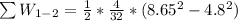
`\sum W_(1-2) =  (1)/(2)  *  (4)/(32) *   (8.65^2  -  4.8^2)`

=>
`W_(1-2) =  3.24 \  ft \cdot lb`