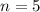Question

A diffraction grating has 300 lines per mm. If light of wavelength 630 nm is sent through this grating, what is the highest order maximum that will appear? A diffraction grating has 300 lines per mm. If light of wavelength 630 nm is sent through this grating, what is the highest order maximum that will appear? 5 6 2 8 5.3A diffraction grating has 300 lines per mm. If light of wavelength 630 nm is sent through this grating, what is the highest order maximum that will appear? A diffraction grating has 300 lines per mm. If light of wavelength 630 nm is sent through this grating, what is the highest order maximum that will appear? 5 6 2 8 5.3

Answer 1

The order of maximum is
`n = 5`

Step-by-step explanation:

From the question we are told that

The diffraction grating is k = 300 lines per mm = 300000 lines per m

The wavelength is
`\lambda  =  630 \  nm  =  630 *10^(-9) \  m`

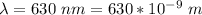
Generally the condition for constructive interference is mathematically represented as

`dsin \theta = n  * \lambda`

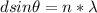
Here n is the order maximum

d is the distance the grating which is mathematically represented as

`d =  (1)/(k)`

=>
`d =  (1)/(300000)`

=>
`d =  3.3*10^(-6)\  m`

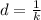
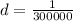
So

`n  = (dsin \theta)/( \lambda)`

at maximum
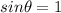
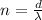
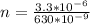
`sin\theta  =  1`

`n  = (d)/(\lambda)`

=>
`n  = (3.3*10^(-6))/(630 *10^(-9))`

=>
`n = 5`