Answer:
Explanation:
Given that:
The null hypothesis:
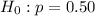
The alternative hypothesis:
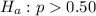
Suppose the number of samples n from the population is 16
The observed value x of the test statistics for testing the hypothesis for this study is x = 14
However, if the null hypothesis is true, then the distribution of the test statistics follows a binomial distribution which is expressed as:
bin(n=16, p = 0.5) distribution
The p-value for this test statistics can be computed as:
p-value = P(X ≥ 14)
p-value = 1 - P (X ≤ 13)
p-value =
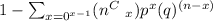
p-value =
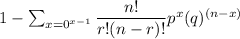
p-value = 0.00209