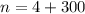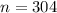Question

Find the value of n so that the line perpendicular to y=−15x − 6 passes through the points (n, −12) and (4, 8) .

Answer 1

`n = 304`

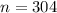
Explanation:

Given

`y = -15x - 6`

`Points: (n,-12) \& (4,8)`

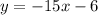
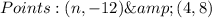
Required

Determine the value of n

First, we need to determine the slope of the given equation.

An equation is of the form;

`y = m_1x + b`

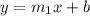
Where m = slope.

By comparison.

`m_1 = -15`

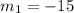
Since the given point and the given equation are perpendicular, then:

`m = -(1)/(m_1)`

`m = -(1)/(-15)`

`m = (1)/(15)`

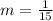
The slope of the point is calculated as thus:

`m = (y_2 - y_1)/(x_2 - x_1)`

Where

`(x_1,y_1) = (n,-12)`

`(x_2,y_2) = (4,8)`

`m = (1)/(15)`

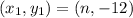
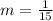
So, we have:

`(1)/(15) = (8 - (-12))/(4 - n)`

`(1)/(15) = (8 +12)/(4 - n)`

`(1)/(15) = (20)/(4 - n)`

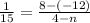
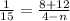
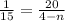
Cross Multiply

`4 - n = 20 * 15`

`4 - n = 300`

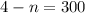
Solve for n

`n = 4 + 300`

`n = 304`