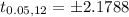Question

g A pharmaceutical manufacturer has been researching new formula to provide quicker relief of minor pains. His laboratories have produced several different formula which he wanted to test. Fifteen people who complained of minor pains were recruited for an experiment. Five were given formula 1, five were given formula 2, and the last five were given formula 3. Each was asked to take the medicine and report (X) the length of time until some relief was felt. Using the results of the output, at 0.05 level of significance, what is the critical value for testing differences in the population mean times for pain relief

Answer 1

Explanation:

From the question we are told that

The sample size is n = 15

The level of significance is
`\alpha = 0.05`

The null hypothesis is
`H_o:\mu_1 = \mu_2 = \mu_3`

The alternative hypothesis is
`H_a : \mu_1 \\e \mu_2 \\e \mu_3`

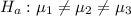
Generally the degree of freedom is mathematically represented as

`df = 5 + 5 + 5 - 3`

=>
`df = 12`

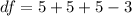
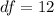
Generally the critical value of
`\alpha = 0.05` at a degree of freedom of
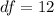`df = 12` is

`t_(0.05 , 12) = \pm 2.1788`