Question

A carpet cleaner charges $59 for a service call and $30 for each room cleaned. A customer does not want to spend more than $125 for having the carpets in his house cleaned. Choose the correct inequality for the situation and the total number of rooms the customer can afford to have cleaned.

Answer 1

No sé exactamente de qué se trata el ejercicio, hay muchas respuestas posibles

Explanation:

Answer 2

`59 + 30c \leq 125`

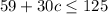
2 rooms

Explanation:

Given

`Base\ Amount = \$59`

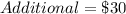`Additional = \$30` per carpet

`Customer\ Budget = \$125`

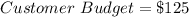
Required

Write an inequality to determine the situation

Represent the number of carpets with c

First, we need to determine an expression for the cleaner's charges:

This is:

`59 + 30 * c`

`59 + 30c`

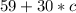
Since the customer's budget do not exceed $125.

This implies that the cleaner's charges must be less than or equal to the budget.

So, we have:

`59 + 30c \leq 125`

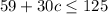
Solving further: [Collect Like Terms]

`30c \leq 125 - 59\\`

`30c \leq 66`

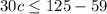
Divide through by 30

`c \leq 66/30`

`c \leq 2.2`

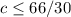
Since number of rooms can't be fraction;

The maximum number of rooms the customer can afford is 2