Answer:
The 10-meter long rod of an SR-71 airplane expands 0.02 meters (2 centimeters) when plane flies at 3 times the speed of sound.
Step-by-step explanation:
From Physics we get that expansion of the rod portion is found by this formula:
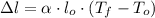 (Eq. 1)
(Eq. 1)
Where:
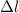 - Expansion of the rod portion, measured in meters.
- Expansion of the rod portion, measured in meters.
 - Linear coefficient of expansion for titanium, measured in
- Linear coefficient of expansion for titanium, measured in
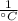 .
.
 - Initial length of the rod portion, measured in meters.
- Initial length of the rod portion, measured in meters.
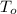 - Initial temperature of the rod portion, measured in Celsius.
- Initial temperature of the rod portion, measured in Celsius.
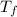 - Final temperature of the rod portion, measured in Celsius.
- Final temperature of the rod portion, measured in Celsius.
If we know that
 ,
,
 ,
,
 and
and
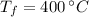 , the expansion experimented by the rod portion is:
, the expansion experimented by the rod portion is:
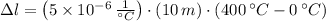

The 10-meter long rod of an SR-71 airplane expands 0.02 meters (2 centimeters) when plane flies at 3 times the speed of sound.