Question

1. An express train, traveling at 36 m/s, is accidentally sidetracked onto a local train track. The express engineer spots a local train 100 m ahead on the same track and traveling in the same direction at a constant 11 m/s. The local engineer is unaware of the situation. The express engineer jams on the brakes and slows the express at 3.0 m/s2. (a) To determine whether the trains collide, use kinematics to calculate their positions when the express train stops: i. How much time will it take the express train to stop? ii. In that time, how far will the express train have moved? iii. In that time, how far will the local train have moved? iv. Based on the results of ii. and iii., do the trains collide? Explain.

Answer 1

(i) 12 seconds

(ii) 216 meters from the initial position

(iii) 132 meters from the initial position

(iv) No

Step-by-step explanation:

Speed of express train =36 m/s

Speed of local train =11 m/s

The initial distance between the local train and passenger train =100 m.

Due to the application of breaks, the express train slows at the rare of
`3.0 m/s^2.`

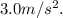
So, the acceleration of the express train,
`a=-3 m/s^2`.

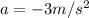(i) Let t be the time the express train takes to stop.

From the equation of motion,

v=u+at

where, v: final velocity, u: initial velocity, a: constant acceleration, t: time taken to change the speed from u to v.

In this case, v=0, u=36 m/s,
`a=-3 m/s^2`

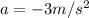
So, 0=36+(-3)t

`\Rightarrow t= 36/3=12` seconds.

(ii) To compute the distance traveled, s, till the express train stops, using

`v^2=u^2+2as`

`\Rightarrow 0^2=36^2+2(-3)s`

`\Rightarrow s=(36*36)/(6)`

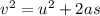
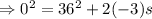
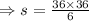
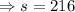`\Rightarrow s=216` meters.

(iii) The local train is moving at a speed of 11 m/s

So, in 12 seconds, the distance, d, traveled by the local train

d= 11x12=132 meters [as distance= speed x time]

(iv) Let 0 be the reference position which is the initial position of the express train.

So, at the initial time, the position of the local train is at 100m.

After 12 seconds:

The position of the express train is at 216 m [using part (ii)]

and the position of the local train is at 100+132=232m [using part (iii)].

So, the local train is still ahead of the express train, hence the trains didn't collide.