Question

C, G, and D are collinear. E, G, and F are also collinear. The two lines

intersect at point G. Line EF bisects line CD. Find EG: if CG=5x-1, GD=7x-
13, EF=6x-4, and GF=13.

Answer 1

EG is 19 units

Explanation:

Let us solve the question

∵ Lines CD and EF intersected at point G

∴ CD = CG + GD

∴ EF = EG + GF

∵ Line EF bisects line CD

→ That means G is the midpoint of CD

∴ CG = GD

∵ CG = 5x -1

∵ GD = 7x - 13

→ Equate them to find x

∴ 7x - 13 = 5x -1

→ Add 13 to both sides

∴ 7x -13 + 13 = 5x - 1 + 13

∴ 7x = 5x + 12

→ Subtract 5x from both sides

∴ 7x - 5x = 5x - 5x + 12

∴ 2x = 12

→ Divide both sides by 2

∴
`(2x)/(2)=(12)/(2)`

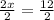
∴ x = 6

∵ EF = 6x - 4

→ Substitute x by 6 to find its length

∴ EF = 6(6) - 4 = 36 - 4

∴ EF = 32

∵ EF = EG + GF

∵ GF = 13

∴ 32 = EG + 13

→ Subtract 13 from both sides

∵ 32 - 13 = EG + 13 - 13

∴ 19 = EG

∴ EG = 19 units