Question

At a birthday party, 5 boys and 3 girls are seated in a row. How many different arrangements are possible if there are no boys between any two girls?

Answer 1

Basic Concept:

Since there are no boys between 2 girls, we will take the 3 girls as 1 single unit and permute the seats

We have 6 slots (5 for boys and 1 for all 3 girls)

We have 6 slots and 6 people to sit on them (taking the 3 girls as one)

Permutations:

So, the total number of seating arrangements is 6P6

which will be equal to 6 factorial (6P6 =
`(n!)/(n! - r!)` =
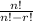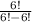`(6!)/(6! - 6!)` = 6! (since 0! = 1))

Total possible seating arrangements = 6!

Total possible seating arrangements = 720