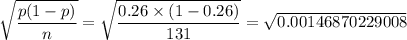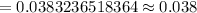Answer: a) Unimodal and symmetric
b) 0.26
c) 0.038
Explanation:
Given: Sample size of investors (n)= 131
True proportion of smartphone users(p) =26%
a) Since sampling distribution for the sample proportion is approximately normal when n is larger.
Normal distribution is Unimodal and symmetric.
So correct option : Unimodal and symmetric
b) mean of this sampling distribution = p = 0.26
c) standard deviation of the samplingdistribution =