Question

A 0.50-kg ball, attached to the end of a horizontal cord, is rotated in a circle of radius 1.9 m on a frictionless horizontal surface. If the cord will break when the tension in it exceeds 85 N, what is the maximum speed the ball can have?

Answer 1

`\boxed {\boxed {\sf 18 \ m/s}}`

Step-by-step explanation:

The ball is moving in a circle, so the force is centripetal.

One formula for calculating centripetal force is:

`F_c= \frac{mv^2}r}`

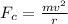
The mass of the ball is 0.5 kilograms. The radius is 1.9 meters. The centripetal force is 85 Newtons or 85 kg*m/s².

• 
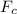  `F_c`= 85 kg*m/s²
• m= 0.5 kg
• r= 1.9 m

Substitute the values into the formula.

`85 \ kg*m/s^2 = (0.5 \ kg *v^2)/(1.9 \ m)`

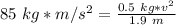
Isolate the variable v. First, multiply both sides by 1.9 meters.

`(1.9 \ m)(85 \ kg*m/s^2) = (0.5 \ kg *v^2)/(1.9 \ m)*1.9 \ m`

`(1.9 \ m)(85 \ kg*m/s^2) = {0.5 \ kg *v^2}`

`161.5 \ kg*m^2/s^2 = 0.5 \ kg*v^2`

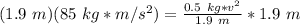
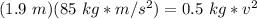
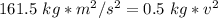
Divide both sides by 0.5 kilograms.

`\frac {161.5 \ kg*m^2/s^2}{0.5 \ kg} = (0.5 \ kg*v^2)/(0.5 \ kg)`

`\frac {161.5 \ kg*m^2/s^2}{0.5 \ kg} =v^2`

`323 \ m^2/s^2 = v^2`

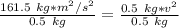
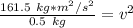
Take the square root of both sides of the equation.

`\sqrt {323 \ m^2/s^2} =\sqrt{ v^2`

`\sqrt {323 \ m^2/s^2} =v`

`17.9722007556 \ m/s =v`

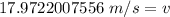
The original measurements have 2 significant figures, so our answer must have the same.

For the number we found, 2 sig fig is the ones place. The 9 in the tenth place tells us to round the 7 to an 8.

`18 \ m/s =v`

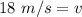
The maximum speed is approximately 18 meters per second.