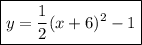Question

Write the equation for a parabola whose vertex is (-6,-1) and passes through (-12,17).

Answer 1

`y=(1)/(2)(x+6)^2-1`

Explanation:

Equation of the Quadratic Function

The vertex form of the quadratic function has the following equation:

`y=a(x-h)^2+k`

Where (h, k) is the vertex of the parabola that graphically represents the function, and a is a coefficient different from zero.

The vertex of the required equation is located at (-6,-1)

The parabola passes through (-12,17)

Substituting the coordinates of the vertex, the equation of the function is:

`y=a(x+6)^2-1`

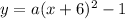
The value of a will be determined by using the given point:

`17=a(-12+6)^2-1`

Operating:

`17=a(36)-1`

`36a=18\Rightarrow a=18/36=1/2`

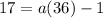
Solving:

`a=1/2`

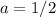
The equation of the parabola is:

`\boxed{y=(1)/(2)(x+6)^2-1}`