Question

Any help with this would be great, I'm not the best at these, and I'm behind from being sick and need to catch up.

Answer 1

Explanation:

Let say the following:

p = number of points

We know that they scored the same number of points in Game 1 and Game 2.

But in Game 3, they scored 6 points less than Game 1 and Game 2.

Therefore,

Game 1 = p

Game 2 = p

Game 3 = p - 6

To find the average you use the following formula:

Number of points / number of games = average

Basically,

(Game 1 + Game 2 + Game 3 ) / 3 = 63

So substitute the equations we made earlier and you get:

`(Game 1 + Game 2 + Game 3)/(3) = 63\\(p + p + (p - 6))/(3) = 63`

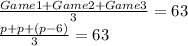
After this you just want to solve for "p"

Multiply both sides by 3 to get rid of the fraction:

p + p + (p - 6) = 63 x 3 = 189

3p - 6 = 189

Add 6 to both sides.

3p = 189 + 6 = 195

Divide both sides by 3

p = 195/3 = 65

So now we know p = 65 points. Substitute this back into our equations for each game:

Game 1 = p , Game 1 = 65 points

Game 2 = p , Game 2 = 65 points

Game 3 = p - 6 , Game 3 = 65 - 6 = 59 points