Question

How do you do this question?

Answer 1

4 Answers: A, B, C, D

=======================================================

Step-by-step explanation:

f(x) is continuous when x >= 1. The only discontinuity for f(x) is when x = 0, but 0 is not part of this interval.

f(x) is positive for any valid x value in the domain since x^6 is always positive. In general, x^n is positive for all x when n is any even number.

f(x) is decreasing. You can see this through a table of values or through a graph. For anything in the form 1/(x^k), it will be a decreasing function because x^k gets larger, so 1/(x^k) gets smaller, when x goes to infinity.

--------------------

The conditions to use the integral test have been met. So we have to see if
`\displaystyle \int_1^(\infty)f(x)dx` converges or not.

Let's integrate and find out

`\displaystyle \int (1)/(x^6) dx = \int x^(-6) dx\\\\\\ \displaystyle \int (1)/(x^6) dx = (1)/(1+(-6))x^(-6+1)+C\\\\\\ \displaystyle \int (1)/(x^6) dx = (1)/(-5)x^(-5)+C\\\\\\ \displaystyle \int (1)/(x^6) dx = -(1)/(5)*(1)/(x^5)+C\\\\\\ \displaystyle \int (1)/(x^6) dx = -(1)/(5x^5)+C\\\\`

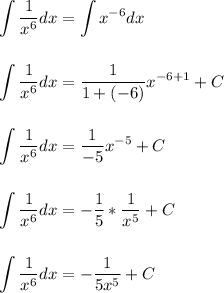
So we have

`\displaystyle g(x) = \int f(x) dx\\\\\\\displaystyle g(x) = \int (1)/(x^6) dx\\\\\\\displaystyle g(x) = -(1)/(5x^5)+C\\\\\\`

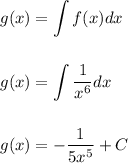
Meaning that,

`\displaystyle \int_(a)^(b) f(x) dx = g(b)-g(a)\\\\\\\displaystyle \int_(a)^(b) (1)/(x^6) dx = \left(-(1)/(5b^5)+C\right)-\left(-(1)/(5a^5)+C\right)\\\\\\\displaystyle \int_(a)^(b) (1)/(x^6) dx = -(1)/(5b^5)+(1)/(5a^5)\\\\\\`

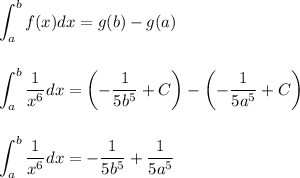
If we plug in a = 1 and apply the limit as b approaches positive infinity, then the expression
`-(1)/(5b^5)+(1)/(5a^5)` will turn into
`(1)/(5)`

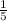
Therefore,

`\displaystyle \int_(1)^(\infty) (1)/(x^6) dx = (1)/(5)\\\\\\`

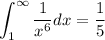
Because this integral converges, this means the series
`\displaystyle \sum_(n=1)^(\infty)(1)/(n^6)` also converges as well by the integral test.