Question

Given B(17,5), C(11, -3), D-1, 2), and
E(x, -6), find the value of x so that BC || DE.

Answer 1

`x=-7`

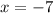
Explanation:

Remember that:

• Two lines are parallel if their slopes are the same.
• Two lines are perpendicular if their slopes are negative reciprocals.
• And two lines are neither (a.k.a intersecting) if they are neither parallel nor perpendicular.

We want to find the value of x such that
`\overline{BC}\parallel\overline{DE}`.

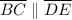Therefore, the slopes of BC and DE must be equivalent.

So, let's find the slope of BC first.

BC)

We can use the slope formula:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

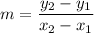
Let B(17, 5) be (x₁, y₁) and let C(11, -3) be (x₂, y₂). Substitute:

`\displaystyle m=(-3-5)/(11-17)`

Subtract:

`m=-8/-6=4/3`

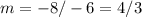
So, the slope of BC is 4/3.

DE)

Let D(-1, 2) be (x₁, y₁) and let E(x, -6) be (x, y₂). Substitute:

`\displaystyle m=(-6-2)/(x-(-1))`

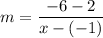
We know that the two slopes must be equal. So, the slope of DE must also be 4/3. Substitute 4/3 for m:

`\displaystyle (4)/(3)=(-6-2)/(x-(-1))`

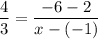
Solve for x. Simplify the right:

`\displaystyle (4)/(3)=(-8)/(x+1)`

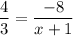
Cross multiply:

`4(x+1)=-8(3)`

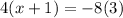
Multiply on the right:

`4(x+1)=-24`

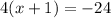
Divide both sides by 4:

`x+1=-6`

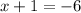
Subtract 1 from both sides:

`x=-7`

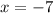
So, the value of x is -7.

And we're done!