Question

Marlina received her salary on Friday. She spent one-half of her salary on rent and then spent $338 on her electric and water bill. Next, she spent one-half of what was left on her phone bill. After spending $56.00 on gas for her car, Marlina had $102.00 left. How much did Marlin spend on rent?

Answer 1

9514 1404 393

Answer:

$654

Explanation:

Let r represent the amount of Marlina's rent. Since that is half her salary, the amount remaining after paying rent is also equal to r. The disposition of that remaining amount is ...

$338 on electric and water (E&W)

(1/2)(r -338) on phone . . . this is half of what is left after paying E&W

$56 on gas

$102 unaccounted

__

The total of these amounts is equal to r, so we have ...

r = 338 + 1/2(r -338) +56 +102

1/2r = 338 -169 +56 +102 = 327 . . . . . eliminate parentheses, subtract 1/2r

r = 2(327) = 654 . . . . . multiply by 2 to get r by itself

Marlina spends $654 on rent.

Answer 2

Marlina spent $654 on rent.

Explanation:

Let the amount of money Marlina's received for her salary be x.

We know that she spent one-half of her salary on rent and then $338 on her electric and water bill. Therefore, the amount of money she has left can be so far can be represented by:

`\displaystyle x-(1)/(2)x-338`

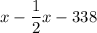
Next, she spent one-half of what was left on her phone bill. So, we can subtract one-half of the above expression to what we have so far.

`\displaystyle x-(1)/(2)x-338-(1)/(2)\left(x-(1)/(2)x-338\right)`

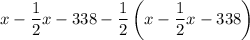
Finally, she spent $56 for gas. Thus, we will subtract 56:

`\displaystyle x-(1)/(2)x-338-(1)/(2)\left(x-(1)/(2)x-338\right)-56`

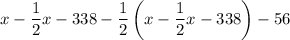
And we know that after all of the expenses, she will have $102 left. Therefore:

`\displaystyle x-(1)/(2)x-338-(1)/(2)\left(x-(1)/(2)x-338\right)-56=102`

We want to know how much Marlina spent on rent. So, we can find her salary, since we know the rent is one-half of the salary. To find the salary, we will solve for x.

Distribute:

`\displaystyle x-(1)/(2)x-338-(1)/(2)x+(1)/(4)x+169-56=102`

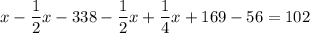
Rearrange:

`\displaystyle \left(x-(1)/(2)x-(1)/(2)x+(1)/(4)x\right)+(-338+169-56)=102`

Combine like terms:

`\displaystyle (1)/(4)x-225=102`

Add 225 to both sides:

`\displaystyle (1)/(4)x=327`

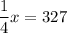
Multiply both sides by 4:

`x=\$ 1308`

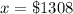
Therefore, Marlina received a total of $1308 for her salary.

We know that she spent half of her salary on rent. Therefore, the amount she spent on rent is:

`\displaystyle r=(1)/(2)(1308)=\$ 654`

Marlina spent a total of $654 on rent.