Question

According to an​ airline, flights on a certain route are on time ​% of the time. Suppose flights are randomly selected and the number of​ on-time flights is recorded. ​(a) Explain why this is a binomial experiment. ​(b) Find and interpret the probability that exactly flights are on time. ​(c) Find and interpret the probability that fewer than flights are on time. ​(d) Find and interpret the probability that at least flights are on time. ​(e) Find and interpret the probability that between and ​flights, inclusive, are on time.

Answer 1

(a) Explained below.

(b) 0.0294

(c) 0.0173

(d) 0.09827

(e) 0.0452

Explanation:

The complete question is:

According to an​ airline, flights on a certain route are on time 80​% of the time. Suppose 25 flights are randomly selected and the number of​ on-time flights is recorded.

​(a) Explain why this is a binomial experiment.

​(b) Find and interpret the probability that exactly 16 flights are on time. ​

(c) Find and interpret the probability that fewer than 16 flights are on time.

​(d) Find and interpret the probability that at least 16 flights are on time.

​(e) Find and interpret the probability that between 14 and ​16 flights, inclusive, are on time.

Solution:

(a)

Let the random variable X be defined as the number of​ on-time flights.

A Binomial experiment has the following properties:

• There are a fixed number of trials (n).
• Each trial are independent of the others.
• Each trial has only two outcomes: Success and Failure
• Each trial has the same probability of success (p).

If a random variable X is used in an experiment and the experiment has all the above mentioned properties, then the random variable X is known as a binomial random variable.

All of these properties can be confirmed for the random variable X.

Thus, this is a binomial experiment.

(b)

Compute the probability that exactly 16 flights are on time as follows:

`P(X=16)={25\choose 16}(0.80)^(16)(0.20)^(25-16)`

`=2042975* 0.0281475* 0.000000512\\=0.029442375072\\\approx 0.0294`

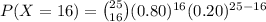
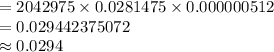
Thus, the probability that exactly 16 flights are on time is 0.0294.

(c)

Compute the probability that fewer than 16 flights are on time as follows:

`P(X<16)=\sum\limits^(15)_(x=0){{25\choose x}(0.80)^(x)(0.20)^(25-x)}`

`=0.0000+0.0000+....+0.011777\\=0.0173`

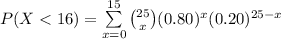
Thus, the probability that fewer than 16 flights are on time is 0.0173.

(d)

Compute the probability that at least 16 flights are on time as follows:

`P(X\geq 16)=1-P(X<16)`

`=1-0.0173\\=0.9827`

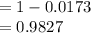
(e)

Compute the probability that between 14 and 16 ​flights, inclusive, are on time as follows:

`P(14\leq X\leq 16)=\sum\limits^(16)_(x=14){{25\choose x}(0.80)^(x)(0.20)^(25-x)}`

`=0.004+0.0118+0.0294\\=0.0452`

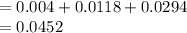
Thus, the probability that between 14 and 16 ​flights, inclusive, are on time is 0.0452.