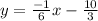Question

Theequationforlineucanbewrittenasy = 6x + 6 . Line v , whichisperpendiculartoline u , includes the point (10, –5) . What is the equation of line v ? Write the equation in slope-intercept form. Write the numbers in the equation as proper fractions, improper fractions, or integers.

Answer 1

`y=(-1)/(6)x-(10)/(3)`

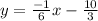
Explanation:

Slope intercept form of the equation of a line is
`y=mx+b` where m denotes the slope of line and
`b` denotes the y-intercept.

Equation of a line u is
`y=6x+6`

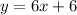
Here, slope of line u = m = 6

As the line v is perpendicular to line u, product of slopes of both the lines is equal to
`-1`.

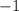Slope of line v
`=(-1)/(6)`

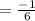
So, slope intercept form of the line v is
`y=(-1)/(6)x+b`

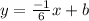
As point
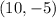`(10,-5)` lies on the line v, put
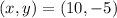`(x,y)=(10,-5)` in equation
`y=(-1)/(6)x+b`

`-5=(-1)/(6)(10)+b\\-5=(-5)/(3)+b\\ -5+(5)/(3)=b\\(-15+5)/(3)=b\\\\(-10)/(3)=b`

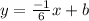
Equation of line v is
`y=(-1)/(6)x-(10)/(3)`