Answer:
y = x - 60
Explanation:
Choose any two pairs/points on the line. In this case, I chose (80, 20) and (40, -20). After that, I plugged them into the following formula for slope:
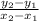
Choose which pair will be pair 2 and which pair will be pair 1. The y's must be always on the top as well and the x's always on the bottom. In this case, I chose (80, 20) to be pair 2 and (40, -20) to be pair 1.
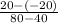
Since a negative and a negative equals a positive, it will be rewritten as:
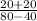
Once you solve it, you should get
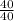 which is basically just 1. Your slope is 1, so plug that into the "m," or the slope, in slope-intercept form: y = mx + b. Since we have a 1 for the slope, it can be shown as an invisible 1 so you can just simply write the x. To find b, the y-intercept, look for the point where the line intersects the y-axis. That point is (0, -60). Therefore, your y-intercept is -60. Now, if you write out the entire thing, you now have y = x - 60.
which is basically just 1. Your slope is 1, so plug that into the "m," or the slope, in slope-intercept form: y = mx + b. Since we have a 1 for the slope, it can be shown as an invisible 1 so you can just simply write the x. To find b, the y-intercept, look for the point where the line intersects the y-axis. That point is (0, -60). Therefore, your y-intercept is -60. Now, if you write out the entire thing, you now have y = x - 60.