Question

The gas cyclobutane, C4H8(g), can be used in welding. When cyclobutane is burned in oxygen, the reaction is: C4H8(g) + 6 O2(g)4 CO2(g) + 4 H2O(g) (a) Using the following data, calculate ΔH° for this reaction. ΔH°f kJ mol-1: C4H8(g) = 27.7 ; CO2(g) = -393.5 ; H2O(g) = -241.8 ΔH° = kJ (b) Calculate the total heat capacity of 4 mol of CO2(g) and 4 mol of H2O(g), using CCO2(g) = 37.1 J K-1 mol-1 and CH2O(g) = 33.6 J K-1 mol-1. C = J K-1 (c) When this reaction is carried out in an open flame, almost all the heat produced in part (a) goes to raise the temperature of the products. Assuming that the reactants are at 25°C, calculate the maximum flame temperature that is attainable in an open flame burning cyclobutane in oxygen. The actual flame temperature would be lower than this because heat is lost to the surroundings. Maximum temperature = °C

Answer 1

a

`\Delta H^o _(rxn) = -2568.9 \  kJ`

b

`H  = 350 JK^(-1)`

c

`T_(max)  = 32.4 ^o C`

Step-by-step explanation:

From the question we are told that

The reaction of cyclobutane and oxygen is

`C_4H_8_((g)) + 6 O_2_((g)) \to 4 CO_2_((g)) + 4 H_2O_((g))`

ΔH°f (kJ mol-1) : C4H8(g) = 27.7 ; CO2(g) = -393.5 ; H2O(g) = -241.8 ΔH° = kJ

Generally ΔH° for this reaction is mathematically represented as

`\Delta H^o _(rxn) = [[4 * \Delta H^o_f (CO_2_((g)) ) + 4 * \Delta H^o_f(H_2O_((g)) ] -[\Delta H^o_f (C_2H_6_((g)) + 6 * \Delta H^o_f (O_2_((g))) ] ]`

=>
`\Delta H^o _(rxn) = [[4 * (-393.5) + 4 * (-241.8) ] -[ 27.7 + 6 * 0]`

=>
`\Delta H^o _(rxn) = -2568.9 \  kJ`

Generally the total heat capacity of 4 mol of CO2(g) and 4 mol of H2O(g), using CCO2(g) = 37.1 J K-1 mol-1 and CH2O(g) = 33.6 J K-1 mol-1. C = J K-1 is mathematically represented as

`H  = [ 4 * C_{CO_2_((g))} + 6* C_{CH_2O_((g))}]`

=>
`H  = [ 4 * 37.1 + 6* 33.6 ]`

=>
`H  = 350 JK^(-1)`

From the question the initial temperature of reactant is
`T_i  =  25^oC`

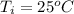
Generally the enthalpy change(
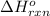`\Delta H^o _(rxn)`) of the reaction is mathematically represented as

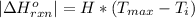
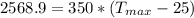
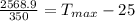
`|\Delta H^o _(rxn) |=  H  * (T_(max) -T_i)`

`2568.9 =   350  * (T_(max) -25)`

=>
`(2568.9 )/(350)  =  T_(max) - 25`

=>
`T_(max)  = 32.4 ^o C`