Question

Jacob is building a square pyramid for a class project. He needs to cover the entire pyramid in aluminum foil. The base of the pyramid has a perimeter of 76 centimeters. The slant height of each triangular side is 28 centimeters. What is the surface area, in square centimeters, of Jacob's pyramid?

Answer 1

The surface area of Jacob's pyramid is 1425cm²

Explanation:

Step 1

Find the dimensions

We will be finding the dimensions to find the surface area. We know on what is the perimeter of the square base, and what is the slant height of each triangle. So that's enough to know, if we know the perimeter, of the square base, we can divide that by 4 to get our 1 side's length. Then, we can calculate the square base's SA and the Triangle's SA!

76/4=19

Now we have our dimensions ready!

Square Base each side length: 19 centimeters

Triangle slant height for each single: 28 centimeters

Step 2

Figure out the SA's now!

Now, let's figure out all the SA's.

First the square base!

19x19=361cm²

Now, let's figure out the SA for all of the triangles

As we know, to find the Area or Surface Area (SA) of each triangle, we have to use this formula:

`SA=(1)/(2) *B*H`

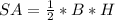
But to find the surface area of 4 triangles at once, we will have to use this formula:

`SA=2*B*H`

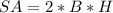
Let's do it now!

`SA=2*19*28\\=2*532\\=1064`

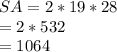
1064cm²

Step 3

Add all the SA's up to get FINAL ANSWER!

Easy peasy lemon squeasy! Let's get on it people!

361+1064=1425cm²

The surface area of Jacob's pyramid is 1425cm²