Answer:
C, D
Explanation:
This equation can be solved any of several ways. One that doesn't require much thought is using the quadratic formula.
For ax² +bx +c = 0, the solutions are ...

In the given equation, a=2, b=11, c=5, so this becomes ...
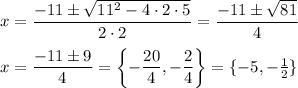
The solutions are ...
C. x = -5
D. x = -1/2
_____
My personal favorite is using a graphing calculator. The solutions are the x-intercepts of the expression on the left. That is, where its value is zero, as the equation says.