Question

The graph of y = ax^2+ C contains the point (2, 14). Which point lies on the graph of y = a(x - 2)² + c?

Answer 1

Given:

The graph of
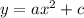`y = ax^2+c` contains the point (2, 14).

To find:

The point which lies on the graph of
`y = a(x-2)^2+c`.

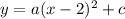Solution:

Consider the given equations are

`y_1 = ax^2+c` ...(i)

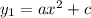`y_2 = a(x-2)^2+c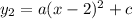` ...(ii)

The translation is defined as

`g(x)=f(x+a)`

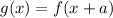
where, a is horizontal shift.

If a>0, then the graph shifts a units left and if a<0, then the graph shifts a units right.

From equation (i) and (ii), it is clear that a=-2. So, graph of
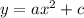`y = ax^2+c` shifts 2 units right to get the graph of
`y = a(x-2)^2+c`.

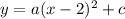It means each point on
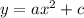`y = ax^2+c` shifts 2 units right.

`(x,y)\to (x+2,y)`

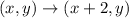
(2, 14) lies on
`y = ax^2+c`.

`(2,14)\to (2+2,14)`

`(2,14)\to (4,14)`

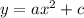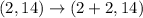
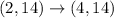
Therefore, (4,14) must be lies on
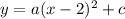`y = a(x-2)^2+c`.