Question

Consider two different traits in mice affecting tail length and fur color. A two-factor cross was made involving true-breeding mice with short tails and white fur to wild-type mice with long tails and brown fur. All F1 offspring had long tails and brown fur. The F1 offspring were then crossed to mice with short tails and white fur. The following phenotypic results were obtained:

118 long tails, brown fur 77 long tails, white fur 114 short tails, white fur 81 short tails, brown fur

Required:
Construct a null hypothesis about these two genes and the expected ratios of the offspring from the cross.

Answer 1

See the answer below

Step-by-step explanation:

Null hypothesis: The two genes are independently assorting and therefore, are not linked to each other.

Let A represents the allele for tail length and B for fur color. Long-tail A is dominant over short tail a while brown fur B is dominant over white fur b.

For the first cross involving true-breeding mice:

AABB x aabb

F1 AaBb (long tails and brown fur)

For the second cross:

AaBb x aabb

4 AaBb - Long-tail, brown fur

4 Aabb - Long tail, white fur

4 aaBb - short tail, brown fur

4 aabb - short tail, white fur

Since the phenotypic ratio from the cross is 1:1:1:1, if the null hypothesis was to be true, it means that the expected phenotype ratio should be 1:1:1:1.

In order to test this hypothesis, we use Chi-square:

phenotype O E X^2

Long-tail, brown fur 118 97.5
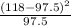`((118-97.5)^2)/(97.5)` = 4.31

Long tail, white fur 77 97.5 4.31

short tail, brown fur 81 97.5 2.79

short tail, white fur 114 97.5 2.79

Total 14.2

Degree of freedom = 4 - 1 = 3

Critical Chi-square value = 7.815

The calculated Chi-square value is more than the critical value, hence, the null hypothesis is rejected.