Answer:
The height of the candle after 14 hours is 15.4 centimeters
Explanation:
A linear function is a polynomial function of the first degree of the form:
f(x)= m*x + b or y= m*x + b
where m is the slope of the function and b is the ordinate at the the y-intercept.
In this case height=m*time + b
Given two points of the line, point 1 (x1, y1) and point 2 (x2, y2), we can find the equation of the line or linear function.
You must first find the slope of the line using the expression:
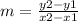
In this case you know the two points are (x1, y1)= (10,17) and (x2, y2)=(21,12.6)
So the slope is:
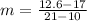
m= -0.4
Now you find the value of b, replacing m in the equation, the values of x and y by the values of the coordinates of one of the points and solving for b to obtain its value.
Replacing (x1, y1)= (10,17) and m=-0.4 in the expresion y=m*x+b:
17= -0.4*10 + b
and solving you get:
17= -4 + b
17 + 4= b
21= b
So: height= -0.4*time + 21 where the height is expressed in centimeters and the time in hours.
To calculate the height of the candle after 14 hours, you simply replace the time by 14:
height= -0.4*14 + 21
height= 15.4
The height of the candle after 14 hours is 15.4 centimeters