Question

Someone please help me

Answer 1

x = -3/2

Explanation:

The removable discontinuity is at the value of x where a denominator factor cancels a numerator factor, and those factors are zero.

`p(x)=(2x+3)/(4x^2-9)=(2x+3)/((2x+3)(2x-3))=(2x+3)/(2x+3)\cdot(1)/(2x-3)\\\\p(x)=(1)/(2x-3)\qquad x\\e-3/2`

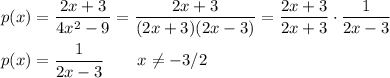
That cancellation occurs where 2x+3 = 0, at x=-3/2.

The function p(x) has a removable discontinuity when x = -3/2.