Question

What value of x makes this equation true?

6x=3x+18

Answer 1

`\rule{50}{1}\large\blue\textsf{\textbf{\underline{Question:-}}}\rule{50}{1}`

What value of x makes the equation
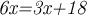`\large\textit{6x=3x+18}` true?

`\rule{50}{1}\large\blue\textsf{\textbf{\underline{Answer and how to solve:-}}}\rule{50}{1}`

`\dagger` When solving an equation, we need to make sure that variables are on one side of the equation, which is usually the left-hand side, and numbers are on the other side, which is usually the right-hand side, of the equation.

`\bigstar` Since we have variables on the right-hand side as well as the left-hand side, we need to move all of them to the left-hand side, with the opposite operation:-

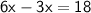`\sf{6x-3x=18}` Subtract 6x-3x:-

`\sf{3x=18}` Now, divide by 3 on both sides:-

`\boxed{\boxed{\sf{x=6}}}\checkmark`

We've found the value of x, but we also need to make sure our solution is correct.

We can do that by substituting 6 for x (into the original equation)

and seeing whether or not we have a true statement:-

`\implies\sf{6(6)=3(6)+18}`

`\implies\sf{36=18+18}`

`\implies\sf{36=36}`

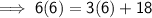
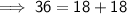
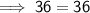
`\bigstar` Since the LHS equals the RHS, our solution (x=6) is correct.

Good luck with your studies.

`\rule{50}{1}\smile\smile\smile\smile\smile\rule{50}{1}`

Answer 2

x= 6

Explanation:

To find the value of x that makes the equation true, first shift all the x terms to one side of the equation.

6x -3x= 18

Simplify:

3x= 18

Divide both sides by 3:

x= 18 ÷3

x= 6