The question is incomplete. Here is the complete question.
The probability density function of the time to failure of an electronic component in a copier (in hours) is

for x > 0. Determine the probability that
a. A component lasts more than 3000 hours before failure.
b. A componenet fails in the interval from 1000 to 2000 hours.
c. A component fails before 1000 hours.
d. Determine the number of hours at which 10% of all components have failed.
Answer: a. P(x>3000) = 0.5
b. P(1000<x<2000) = 0.2325
c. P(x<1000) = 0.6321
d. 105.4 hours
Explanation: Probability Density Function is a function defining the probability of an outcome for a discrete random variable and is mathematically defined as the derivative of the distribution function.
So, probability function is given by:
P(a<x<b) =
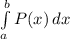
Then, for the electronic component, probability will be:
P(a<x<b) =

P(a<x<b) =
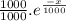
P(a<x<b) =

a. For a component to last more than 3000 hours:
P(3000<x<∞) =
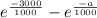
Exponential equation to the infinity tends to zero, so:
P(3000<x<∞) =

P(3000<x<∞) = 0.05
There is a probability of 5% of a component to last more than 3000 hours.
b. Probability between 1000 and 2000 hours:
P(1000<x<2000) =
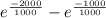
P(1000<x<2000) =
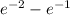
P(1000<x<2000) = 0.2325
There is a probability of 23.25% of failure in that interval.
c. Probability of failing between 0 and 1000 hours:
P(0<x<1000) =
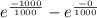
P(0<x<1000) =
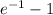
P(0<x<1000) = 0.6321
There is a probability of 63.21% of failing before 1000 hours.
d. P(x) =

0.1 =
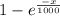
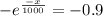
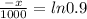
-x = -1000.ln(0.9)
x = 105.4
10% of the components will have failed at 105.4 hours.