Answer:
Option c (21.4%) is the right approach.
Step-by-step explanation:
As we know.
- wA and wB = weights of the securities
- SDA and SDB = standard deviations
- Cor(A,B) = correlation coefficient.
On applying the formula:
⇒
![SD \ Portfolio = [wA^2* SDA^2+wB^2* SDB^2+2* wA* wB* SDA* SDB* Cor(A,B)]^(0.5)](https://img.qammunity.org/2021/formulas/business/college/3x6e3iwl9dj1h8o0bxmv8pfltt71er0unr.png)
On substituting the values, we get
⇒

⇒
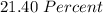 (%)
(%)