Answer:
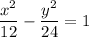
Explanation:
Since the directrices are vertical lines:
- Use the hyperbola equation
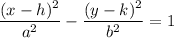
- Foci are
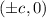

 and
and
 are half the lengths of the tranverse and conjugate axes respectively
are half the lengths of the tranverse and conjugate axes respectively- Directrices are located at the lines
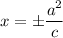
- We assume the center to be
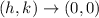
Since we already know our directrices are at the lines
 and we know that
and we know that
 from our foci, we can solve for the value of
from our foci, we can solve for the value of
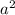 :
:
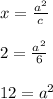
This allows us to solve for the value of
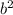 :
:
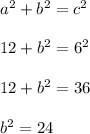
Thus, our equation for the hyperbola is
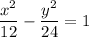 .
.