Question

An object with mass of 1 kg, specific heat of 1000 J/kg°C, surface area of 0.01 m2, and initial temperature of 25°C is placed in a microwave oven with internal temperature of 25°C and heat transfer coefficient of 40 W/m2°C.If the microwave heat input into the object is 50 W,

a) Show that the differential equation that governs the temperature change forthis object is
dT/dt+0.0004T = 0.06
b) Solve this differential equation and find out what the temperature of this object is after 200 seconds heating inside the microwave.

Answer 1

a) True. The differential equation is
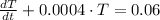`(dT)/(dt)+0.0004\cdot T = 0.06`, where
`T` is measured in Celsius.

b) The temperature of the object after 200 second inside the microwave is approximately 34.611 ºC.

Explanation:

a) The object is heated up by convection and radiation, from First Law of Thermodynamics we get that energy interactions on the object are represented by:

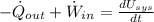`-\dot Q_(out) + \dot W_(in) = (dU_(sys))/(dt)` (Eq. 1)

Where:

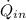`\dot Q_(in)` - Heat transfer rate to the object, measured in watts.

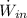`\dot W_(in)` - Electric power given by the microwave owen, measured in watts.

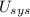`U_(sys)` - Internal energy of the object, measured in watts.

Then, we expand the expression by means of definition from Heat Transfer and Thermodynamics:

`-h_(c)\cdot A_(s)\cdot (T-T_(\infty))+\dot W_(in) = m\cdot c\cdot (dT)/(dt)`

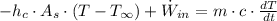
Where:

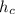`h_(c)` - Heat transfer coefficient, measured in watts per square meter-Celsius.

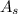`A_(s)` - Surface area of the object, measured in square meters.

`T` - Temperature of the object, measured in Celsius.

`T_(\infty)` - Internal temperature of the microwave oven, measured in Celsius.

`m` - Mass of the object, measured in kilograms.

`c` - Specific heat of the object, measured in joules per kilogram-Celsius.

After some algebraic handling, we get this non-homogeneous first order differential equation:

`(dT)/(dt) = -(h_(c)\cdot A_(s))/(m\cdot c) \cdot (T-T_(\infty)) + (\dot W_(in))/(m\cdot c)`

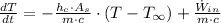
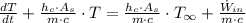`(dT)/(dt)+(h_(c)\cdot A_(s))/(m\cdot c)\cdot T =(h_(c)\cdot A_(s))/(m\cdot c)\cdot T_(\infty)+(\dot W_(in))/(m\cdot c)` (Ec. 2)

If we know that
`h_(c) = 40\,(W)/(m^(2)\cdot ^(\circ)C)`,
`A_(s) = 0.01\,m^(2)`,
`m = 1\,kg`,
`c = 1000\,(J)/(kg\cdot ^(\circ)C)`,
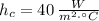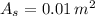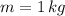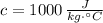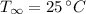`T_(\infty) = 25\,^(\circ)C` and
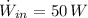`\dot W_(in) = 50\,W`, the differential equation is:

`(dT)/(dt)+0.0004\cdot T = 0.06`

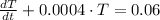
b) The solution of this differential equation is:

`T(t) = T_(o)\cdot e^(-0.0004\cdot t)+ (0.06)/(0.0004)\cdot (1-e^(-0.0004\cdot t))`

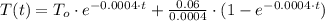
Where
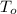`T_(o)` is the initial temperature of the object, measured in Celsius.

`T(t) = T_(o)\cdot e^(-0.0004\cdot t)+150\cdot (1-e^(-0.0004\cdot t))`

`T(t) = 150+(T_(o)-150)\cdot e^(-0.0004\cdot t)`

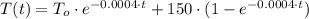
Where
`t` is the time, measured in seconds.

If we know that
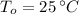`T_(o) = 25\,^(\circ)C`, then:

`T(t) = 150-125\cdot e^(-0.0004\cdot t)` (Ec. 3)

For
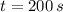`t = 200\,s`, we get that temperature of the object is:

`T(200) = 150-125\cdot e^(-0.0004* 200)`

`T(200) \approx 34.611\,^(\circ)C`

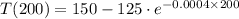
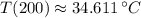
The temperature of the object after 200 second inside the microwave is approximately 34.611 ºC.