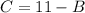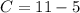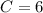Question

Scarborough High School ordered several replacement books for the mathematics department. When the box of books arrived at the high school, it contained Algebra I, Geometry, and Algebra II textbooks. A label on the box reads: "Contents: 23 books, Weight: 93 lbs." An Algebra I book weighs 4 pounds, a Geometry book weighs 3 pounds, and an Algebra II book weighs 5 pounds. The number of Geometry books and Algebra II books combined is one less than the number of Algebra I books.

Answer 1

Algebra 1 = 12 books

Algebra 2 = 5 books

Geometry = 6 books

Explanation:

Given

Represent

Algebra 1 with A

Algebra 2 with B

Geometry with C

For the quantity

`A + B + C = 23`

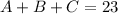
To represent the weight, we have that:

`A = 4lb`

`B = 5lb`

`C = 3lb`

`Total = 93lb`

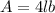
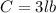
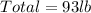
This gives:

`4A + 3B + 5C = 93`

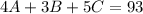
The last sentence in the question can be represented with:

`C + B = A - 1`

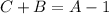
So, the expressions to work with are

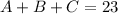`A + B + C = 23` --- (1)

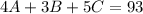`4A + 3B + 5C = 93` --- (2)

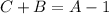`C + B = A - 1` --- (3)

Substitute A - 1 for B + C in (1)

`A + B + C = 23`

`A + A - 1 = 23`

`2A - 1 = 23`

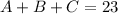
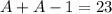
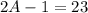
Solve for 2A

`2A = 23 + 1`

`2A = 24`

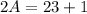
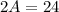
Solve for A

`A = 12`

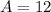
Substitute 12 for A in the (2) & (3)

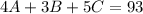
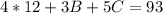
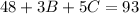
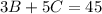`4A + 3B + 5C = 93`

`4 * 12 + 3B + 5C = 93`

`48 + 3B + 5C = 93`

`3B + 5C = 93 - 48`

`3B + 5C = 45` ---- (4)

`C + B = A - 1`

`C + B = 12 - 1`

`C + B = 11`

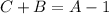
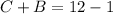
Make C the subject

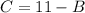`C = 11 - B` ----- (5)

Substitute 11 - B for C in (4)

`3B + 5C = 45`

`3B + 5(11 - B) = 45`

`3B + 55 - 5B = 45`

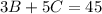
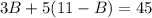
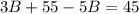
Collect Like Terms

`3B - 5B = 45 - 55`

`-2B = -10`

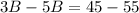
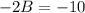
Solve for B

`B = -10/-2`

`B = 5`

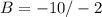
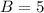
Substitute 5 for B in (5)

`C = 11 - B`

`C = 11- 5`

`C = 6`