Answer:
Both charges are of the same sign (positive or negative) and with a value of 3.73 ηc.
Step-by-step explanation:
Coulomb's Law
The electrical force between two charged objects is directly proportional to the product of their charges and inversely proportional to the square of the distance between the two objects.
Written as a formula:
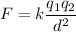
Where:
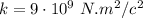
q1, q2 = the objects' charge
d= The distance between the objects
We know both charges are identical, i.e. q1=q2=q. Since the force is repulsive, both charges are positive or negative.
We also are given the force between them:
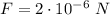
And also the distance
d=25 cm = 0.25 m
Substituting all into the formula:
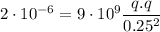
The product of
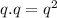 . Operating:
. Operating:
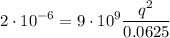
Operating:
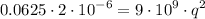
Solving for q:

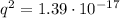
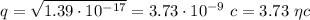
Both charges are of the same sign (positive or negative) and with a value of 3.73 ηc.