Answer:
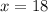
Explanation:
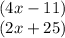
According to the Alternate Exterior Angles Theorem, "when two lines are cut by a transversal, the resulting alternate exterior angles are congruent."
This means that the two expressions above are to be set equal to each other because they are representative of two alternate exterior angles, which are congruent:
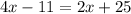
Add
 to both sides of the equation:
to both sides of the equation:
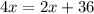
Subtract
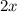 from both sides of the equation:
from both sides of the equation:
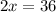
Divide both sides of the equation by the coefficient of
 , which is
, which is
 :
:
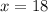
~
Check your work by substituting
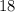 for
for
 in the initial equation:
in the initial equation:
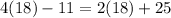
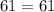
It's correct!