Question

Jason had three more than four times the amount of money that Jeff has. Together they have $72. How much money does each person have?

Answer 1

Jason has $58.20 and Jeff has $13.80.

Explanation:

Let x represent the amount of money Jason has, and y represent the amount of money Jeff has.

We know that together they have $72. So, we can write the following equation:

`x+y=72`

We know that Jason has three more than four times the amount of money Jeff has. So:

`x=3+4y`

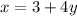
This is a system of equations. We can solve it by using substitution. Let's substitute the second equation into the first. This yields:

`(3+4y)+y=72`

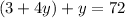
Combine like terms:

`5y+3=72`

Subtract 3 from both sides:

`5y=69`

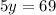
Divide both sides by 5:

`y=69/5=\$13.80`

So, Jeff has $13.80

We know that Jason has three more than four times the amount of money Jeff has.

Therefore, Jason has:

`x=13.8(4)+3`

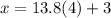
Multiply and add:

`x=\$58.20`

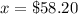
So, Jason has $58.20 and Jeff has $13.80.

And we're done!