Question

What is x and y shown in the photo above?

Answer 1

Y=3

X=11

Explanation:

9x + 25 is = to 13x - 19 so you would do exactly that.

9x + 25 = 13x -19

-9x -9x

25 = 4x -19

+19 +19

44 = 4x

4 4

11 = x

9*11+25= 124

And 124 is not equal to 17y+5 so the equation would be

124 + 17y +5 = 180

124 + 5 = 130

129 + 17y = 180

-129 -129

17y = 51

17 17

Y= 3

Answer 2

`\huge\boxed{x = 11,\ y = 3}}`

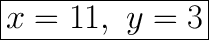
Explanation:

In order to find the value of x and y in this, we have to note a couple of angle relationships.

We know that angles
`13x-19` and
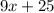`9x+25` are the same because they are corresponding angles. This means that when a line intersects two parallel lines, the angles formed are congruent to the second line.

This means both expressions for the line will be equal to each other:

`13x-19 = 9x+25`

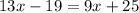
We can now solve for x.

Add 19 to both sides:

`13x = 9x+44`

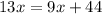
Subtract 9x from both sides:

`4x=44`

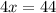
Divide both sides by 4:

`x=11`

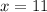
Now that we know that x = 11, we can use another angle relationship to find y.

We know that
`13x-19` and
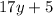`17y+5` are supplementary angles. This means their angle measurements add up to 180°.

Since we know the value of x, we can find the measure of the angle
`13x-19`.

`13(11) -19\\\\143-19\\\\124`

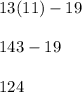
So the
`13x-19` angle is equal to 124 degrees. Since this and
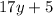`17y+5` are supplementary, that means
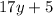`17y+5` must be equal to
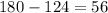`180-124=56` degrees.

`17y+5=56`

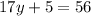
Subtract 5 from both sides:

`17y=51`

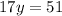
Divide both sides by 17:

`y=3`

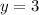
So, x = 11 and y = 3.

Hope this helped!