Answer:
Considering first question
Generally the coefficient of performance of the air condition is mathematically represented as
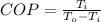
Here
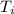 is the inside temperature
is the inside temperature
while
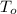 is the outside temperature
is the outside temperature
What this coefficient of performance represent is the amount of heat the air condition can remove with 1 unit of electricity
So it implies that the air condition removes
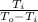 heat with 1 unit of electricity
heat with 1 unit of electricity
Now from the question we are told that the rate at which heat enters an air conditioned building is often roughly proportional to the difference in temperature between inside and outside. This can be mathematically represented as

=>
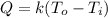
Here k is the constant of proportionality
So
since 1 unit of electricity removes
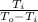 amount of heat
amount of heat
E unit of electricity will remove
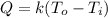
So
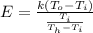
=>
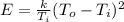
given that
 is constant
is constant
=>
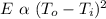
From this above equation we see that the electricity required(cost of powering and operating the air conditioner) is approximately proportional to the square of the temperature difference.
Considering the second question
Assuming that

and
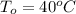
Hence
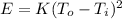
Here K stand for a constant
So
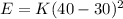
=>
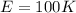
Now if the

Then
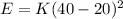
=>
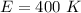
So from this see that the electricity require (cost of powering and operating the air conditioner)when the inside temperature is low is much higher than the electricity required when the inside temperature is higher
Considering the third question
Now in the case where the heat that enters the building is at a rate proportional to the square-root of the temperature difference between inside and outside
We have that
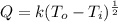
So
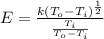
=>
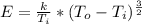
Assuming
 is a constant
is a constant
Then
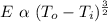
From this above equation we see that the electricity required(cost of powering and operating the air conditioner) is approximately proportional to the square root of the cube of the temperature difference.