Question

I need help please somebody answer ASAP please I’d highly appreciate it

Answer 1

w = 3

Step-by-step explanation:

(6x-12)/3 + 4 = 18/x

Simplify fraction

2x -4 +4 = 18/x

Combine -4+4=0 Divide by 2 to reduce

x = 9/x

Multiply both sides by x

x² = 9

x = 3, -3

Answer 2

2.(b) w= 3

3. x= -3 or x= 3

Explanation:

2.(b) Method 1:

`(2w - 1)/(5) + w + 2 = 2w \\ (2w - 1)/(5) = 2w - w - 2 \\`

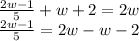
Simplify:

`(2w - 1)/(5) = w - 2`

×5 on both sides:

`2w - 1 = 5(w - 2)`

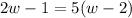
Expand:

`2w - 1 = 5w - 10`

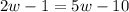
Bring constants to 1 side, w terms to the other:

`5w - 2w = 10 - 1`

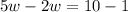
Simplify:

`3w = 9`

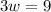
Divide both sides by 3:

`w = 9 / 3 \\ w = 3`

Method 2:

`(2w - 1)/(5) + w + 2 = 2w`

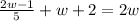
Multiply by 5 throughout:

`2w - 1 + 5(w + 2) = 5(2w)`

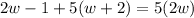
Expand:

`2w - 1 + 5w + 10 = 4w`

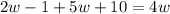
Simplify:

`7w - 9 = 4w`

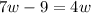
+9 on both sides:

`7w = 4w + 9`

-4w on both sides:

`7w - 4w = 9`

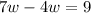
Simplify:

`3w = 9`

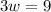
Divide by 3 on both sides:

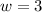
`w = 3`

3.
`(6x - 12)/(3) + 4 = (18)/(x)`

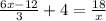
Multiply by 3x on both sides:

`(3x(6x - 12))/(3) + 3x(4) = (3x(18))/(x) \\ x(6x - 12) + 12x = 3(18)`

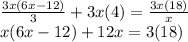
Expand:

`x(6x) - x(12) + 12x = 54 \\ 6x^(2) - 12x + 12x = 54 \\`

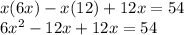
Simplify:

`6 {x}^(2) = 54`

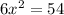
Divide by 6 on both sides:

`{x}^(2) = 9`

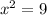
-9 on both sides:

`{x}^(2) - 9 = 0`

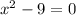
Factorise:

*Since a²-b²= (a +b)(a -b),

`{x}^(2) - {3}^(2) = 0 \\ (x + 3)(x - 3) = 0`

Thus,

x +3= 0 or x -3= 0

x= -3 or x= 3