Question

What is the ratio for the volumes of two similar spheres, given that the ratio of their radii is 5:6?

Answer 1

Given ratio:
`(\text{Radius of Sphere}_1 : \text{Radius of Sphere}_2) = (5 :6)`

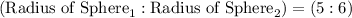
Step-1) Multiply both sides of the ratio by x:

`\implies (\text{Radius of Sphere}_1 : \text{Radius of Sphere}_2) = (5x :6x)`

`\implies \text{Radius of Sphere}_1 = 5x;\ \text{Radius of Sphere}_2 = 6x`

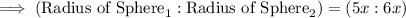
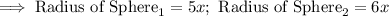
Step-2) Substitute the radiuses of each sphere into the "Volume formula"

Volume of sphere₁ Volume of sphere₂

`\implies \ \ \ \ \ \ \ \ (4)/(3) \pi r^(3) \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ (4)/(3) \pi r^(3)`

`\implies \ \ \ \ \ \ \ \ (4)/(3) \pi (5x)^(3) \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ (4)/(3) \pi (6x)^(3)`

`\implies \ \ \ \ \ \ \ \ (4)/(3) \pi (5x)(5x)(5x) \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ (4)/(3) \pi (6x)(6x)(6x)`

`\implies \ \ \ \ \ \ \ \ (4)/(3) \pi (125x^(3) ) \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ 4 \pi (2x)(6x)(6x)`

`\implies \ \ \ \ \ \ \ \ (500x^(3) )/(3) \pi \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ 288x^(3) \pi`

Step-3) Plug the volume of both spheres in ratio form:

The ratio for the volumes of the two spheres must be in the respective place of the ratio for the radiuses of the two similar spheres. Therefore,

Ratio form:
`\underline{(\small\text{Volume of sphere}_1 : \text{Volume of sphere}_2)}`

`\implies (500x^(3) )/(3) \pi: 288x^(3) \pi`

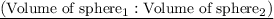
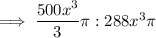
Step-4) Simplify both sides of the ratio:

`\implies (500x^(3) )/(3): 288x^(3)`

`\implies (500 )/(3): 288`

`\implies (500 )/(3)} /{(288)/(1) } \implies (500)/(3) * (1)/(288) \implies (500)/(864) \implies \boxed{(125)/(216)}`

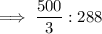
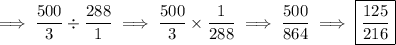
Therefore, the ratio for the volumes of two similar spheres is 125 : 216.

Answer 2

125 : 216

Explanation:

Let the volumes of the spheres be V₁ and V₂ respectively.

Volume (Sphere radius = 5) :

• V₁ = 4/3π(5)³
• V₁ = 4/3π x 125
• V₁ = 500/3π

Volume (Sphere radius = 6) :

• V₂ = 4/3π(6)³
• V₂ = 4/3π x 216
• V₂ = 864/3π

Taking the ratio of V₁ and V₂ :

• V₁/V₂
• 500/3π x 3π/864
• 500/864
• 125 : 216