Question

Question number 8. Prove that 3 + √5 is an irrational number.​

Answer 1

Let's assume the opposite of the statement i.e., 3 + √5 is a rational number.

`\\ \sf \: 3 + √(5) = (a)/(b) \\ \\ \qquad \: \tiny \sf{(where \: \: a \: \: and \: \: b \: \: are \: \: integers \: \: and \: \: b \: \\eq \: 0)} \\`

`\\ \sf \: √(5) = (a)/(b) - 3 = (a - 3b)/(b) \\`

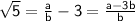
Since, a, b and 3 are integers. So,

`\\ \sf \: (p - 3b)/(b) \\ \\ \qquad \tiny \sf{ \: (is \: \: a \: \: rational \: \: number \:) } \\`

Here, it contradicts that √5 is an irrational number.

because of the wrong assumption that 3 + √5 is a rational number.

`\\`

Hence, 3 + √5 is an irrational number.