Question

Find an equation of the line described below. Write the equation in slope-intercept form (solved for y), when possible.

Through (15,3) and (3,15)

Answer 1

y=-x+18

Explanation:

Equation of a line

A line can be completely defined by two points. Suppose we know the line passes through points A(x1,y1) and B(x2,y2).

The equation for a line can be written as:

`y=mx+b`

Where m is the slope and b is the y-intercept. Both values can be determined by using the coordinates of the given points.

First, determine the slope with the equation:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

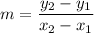
The points are: A(15,3) B(3,15)

`\displaystyle m=(15-3)/(3-15)=(12)/(-12)=-1`

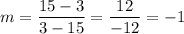
The equation of the line can be written as:

`y=-x+b`

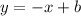
Now, use any point to determine the value of b. Substitute (15,3):

`3=-15+b`

Solve for b:

b=18

The equation of the line is

y=-x+18

The slope is -1 and the y-intercept is 18.