Question

4. An object is thrown vertically upward with a speed of 25 m/s. How much time passes before it

comes back down at 15 m/s? (Air resistance is negligible.)
a. 1.0 S
d. 18 s
b. 4.1 s
e. 27 s
c. 9.85

Can you also explain how you got the answer please?​

Answer 1

Option b is correct: 4.1 s

Step-by-step explanation:

Vertical Launch

An object launched thrown vertically upward where air resistance is negligible, reaches its maximum height in a time t, given by the equation:

`\displaystyle t=(v_o)/(g)\qquad\qquad[1]`

Where vo is the initial speed and g is the acceleration of gravity g=9.8
`m/s^2`.

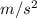Once the object reaches that point, it starts a free-fall motion, whose speed is (downward) given by:

`v_f=g.t\qquad\qquad[2]`

The object considered in the question is thrown with vo=25 m/s. The time taken to reach the maximum height is given by [1]:

`\displaystyle t=(25)/(9.8)=2.551\ sec`

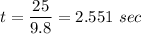
The object starts its falling motion and at some time, it has a speed of vf=15 m/s. Let's find the time by solving [2] for t:

`\displaystyle t=(15)/(9.8)=1.531\ sec`

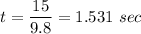
The total time taken by the object to go up and down is

`t_t=2.551\ s+1.531\ s=4.081\ s`

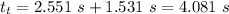
a. This option is incorrect because it's far away from the answer.

d. This option is incorrect because it's far away from the answer.

b. This option is correct because it's a good approximation to the calculated answer.

e. This option is incorrect because it's far away from the answer.

c. This option is incorrect because it's far away from the answer.