Final Answer:
The number of terms in the arithmetic progression (AP) is 19.
Step-by-step explanation:
An arithmetic progression is defined by its first term
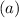 , common difference
, common difference
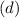 , and last term
, and last term
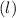 . In this case, the first term
. In this case, the first term
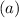 is 13, the common difference
is 13, the common difference
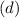 is -2 1/4, and the last term
is -2 1/4, and the last term
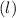 is -23.
is -23.
The formula to find the
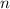 -th term
-th term
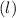 in an arithmetic progression is given by:
in an arithmetic progression is given by:
![\[ l = a + (n-1)d \]](https://img.qammunity.org/2021/formulas/mathematics/college/wh21jn4l9x8gurr59peab2q3su32o7i41a.png)
We are given that
 . Substituting these values into the formula, we get:
. Substituting these values into the formula, we get:
![\[ -23 = 13 + (n-1) \left(-2 (1)/(4)\right) \]](https://img.qammunity.org/2021/formulas/mathematics/college/7chi1vcr8v2wbr77br6rv4orstomygjrbe.png)
Now, solve for
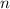 :
:
![\[ -23 = 13 - 2n + (9)/(4) \]](https://img.qammunity.org/2021/formulas/mathematics/college/ty990p3o43utlxijmd0gjgwmrbhommb403.png)
![\[ -36 = -2n + (9)/(4) \]](https://img.qammunity.org/2021/formulas/mathematics/college/z2yz6qw7lshyk8bke4sr4wy118nr3tupjd.png)
![\[ -(147)/(4) = -2n \]](https://img.qammunity.org/2021/formulas/mathematics/college/pyk4rewnfyvh9i4ho7ijmxayuavocrn5r5.png)
Divide both sides by
 to solve for
to solve for
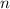 :
:
![\[ (147)/(8) = n \]](https://img.qammunity.org/2021/formulas/mathematics/college/99vuap434w2d042on5012kg2f54it8z5r7.png)
So,
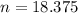 . However, the number of terms
. However, the number of terms
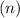 must be a whole number since it represents the count of terms in the sequence. Therefore, we round up to the nearest whole number, and the number of terms in the arithmetic progression is 19.
must be a whole number since it represents the count of terms in the sequence. Therefore, we round up to the nearest whole number, and the number of terms in the arithmetic progression is 19.
In conclusion, the AP has 19 terms. This means that the sequence starts at 13, decreases by -2 1/4 for 18 times, and reaches -23 at the end of the progression.