Question

If the point (a, b) is equidistant from the points (-a, 2) and (2, -b), then prove that 3(a+b)+4=0​

Answer 1

Answer: see proof below

Explanation:

Since (a, b) is equidistant from (-a, 2) and (2, -b), then it is the midpoint of the those two points. Use Midpoint formula to find (a, b).

`M_x=(x_1+x_2)/(2)\qquad \qquad \qquad M_y=(y_1+y_2)/(2)\\\\\\a=(-a+2)/(2)\qquad \qquad \qquad \quad b=(2-b)/(2)\\\\\\2a=-a+2\qquad \qquad \qquad \quad 2b=2-b\\\\\\3a=2\qquad \qquad \qquad \qquad \qquad 3b=2\\\\\\a=(2)/(3)\qquad \qquad \qquad \qquad \qquad b=(2)/(3)`

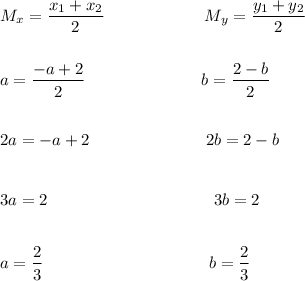
3(a + b) - 4 = 0

`3\bigg((2)/(3)+(2)/(3)\bigg)-4=0\\\\\\3\bigg((4)/(3)\bigg)-4=0\\\\\\4-4=0\\\\0=0\qquad \text{TRUE!}`

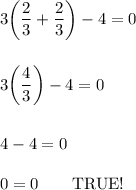
Notice that I changed the equation to "negative 4" because the equation you provided did not make a true statement.