Answer:
Approximately
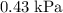 (rounded to two significant figures.)
(rounded to two significant figures.)
Step-by-step explanation:
Let
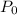 .
.
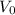 , and
, and
 denote the initial pressure, volume, and temperature of this sample. Let
denote the initial pressure, volume, and temperature of this sample. Let
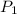 .
.
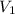 , and
, and
 denote the final pressure, volume, and temperature of this sample.
denote the final pressure, volume, and temperature of this sample.
Convert the two temperatures to degrees Kelvins:
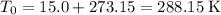 .
.
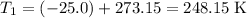 .
.
Based on the assumption that the ammonia sample here is an ideal gas, it should satisfy the ideal gas equation:
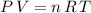 , where:
, where:
 is the pressure of this gas.
is the pressure of this gas.
 is the volume of this gas.
is the volume of this gas.
 is the number of moles of gas particles in this sample.
is the number of moles of gas particles in this sample.
 is the ideal gas constant, and
is the ideal gas constant, and
 is the temperature of this gas in degrees Kelvins.
is the temperature of this gas in degrees Kelvins.
The value of the ideal gas constant,
 , should stay the same during this change.
, should stay the same during this change.
On the other hand, assume that this sample did not leak during this change. Hence, the number of moles of gas particles in the sample,
 , should also stay the same during this change.
, should also stay the same during this change.
Therefore:
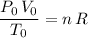 .
.
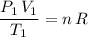 .
.
Combine these two equations to obtain:
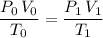 .
.
Rearrange this equation and solve for
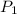 , the final pressure of this sample:
, the final pressure of this sample:
 .
.
The question stats that the Initial pressure of this sample is
 .
.
On the other hand, volume "increased by
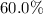 " means that the final volume would be
" means that the final volume would be
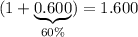 times the initial volume. Therefore:
times the initial volume. Therefore:
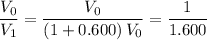 .
.
Solve for the final pressure of this sample,
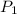 :
:
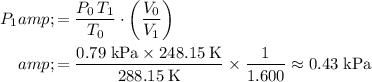 .
.
(Rounded to two significant digits.)