Answer:
1)
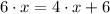 , 2) The value of
, 2) The value of
 is 3, 3) The perimeter and the area of the rectangule are 18 feet and 18 square feet, respectively.
is 3, 3) The perimeter and the area of the rectangule are 18 feet and 18 square feet, respectively.
Explanation:
Let be a rectangle with a length of
 feet and a width of 3 feet. The area (
feet and a width of 3 feet. The area (
 ), measured in square feet, and perimeter (
), measured in square feet, and perimeter (
 ), measured in feet, formulas are, respectively:
), measured in feet, formulas are, respectively:
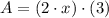
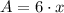 (Eq. 1)
(Eq. 1)
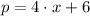 (Eq. 2)
(Eq. 2)
1) Now, we assume that
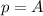 , then we reduce the system of linear equation into a sole linear equation:
, then we reduce the system of linear equation into a sole linear equation:
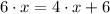 (Eq. 3)
(Eq. 3)
2) We proceed to solve for
 :
:
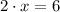

The value of
 is 3.
is 3.
3) Lastly, we obtain the values of the perimeter and area:
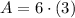
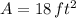

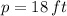
The perimeter and the area of the rectangule are 18 feet and 18 square feet, respectively.